Sunday, February 28, 2010
5 WAYS TO IMPROVE MULTIPLICATION
Below are five outstanding and helpful ways to help kids of grades to practice their multiplication skills based on multiplication tables, so that they can lead in various math multiplication projects.
Five Fun Ways to Practice Multiplication Skills:
Activity 1: Memory Game
Kids mostly opt to play multiplication memory game. They can create their own multiplication memory game focusing on a definite multiplication table. Here, in this example, you we will use table of 3.
Multiplication is a sequential function. You can select a color of construction sheet, cut 2-inch squares, and write the problem (0 x 3, 1 x 3, 2 x 3, etc.). Now, choose one more color of construction sheet, and cut squares and write down the answers (0, 3, 6, etc.).
Next, turn almost all the cards downwards and play the usual memory game. Try to choose a "problem" color and an "answer" color for it. If your memorization is correct, then note down the points and try again. You can do this with almost every set of table.
Activity 2: Use of Internet
Next, parents and teachers may make use of the Internet to help practice multiplication skills for kids. You can find tons of web sites devoted to help kids to practice and polish their entire math skills. They also offer fun and innovative interactive games.
Generally, children tend to practice hard in multiplication problems to improve the game, and when it becomes fun to play, they hardly realize that they are practicing and learning.
Activity 3: Musical Practice
Another helpful way to practice multiplication is to memorize musically. Teachers and parents can find several knowledgeable videos, songs, and recordings, based entirely on multiplication tables and skills.
Several teachers affirm that music is by far the most helpful way to practice multiplication skills.
Activity 4: Single Calculations
1 x anything = itself
You can start such multiplication concept with a few small numbers, for example 7 x 1. Try to stick with small digits until you find the concept easy and simply repeat the number that you are supposed to multiply. For students of higher grades, try for example 283 x 1 along with some fractions.
Activity 5: Doubles Calculations
2 x a number = doubles
Two times a number signifies to add it twice. Thus, those are the doubles in calculation. Tables of two are just the doubles. You can evaluate the doubles, with multiplication equation, but try to explain it in addition form.
For example, 2 x 6 = 12, and in addition, doubles of six is the same 6 + 6 = 12. You can write the solution to the addition problem as the solution to the multiplication problem. Hence, kids need to understand that 2 x n is n + n.
Final Review:
The implementation of this system is very much simple. You can assess the concept of 1 x anything, with varied set of numbers bearing the multiplication tables in mind.
Kids need to observe that, they are not only learning a whole set of multiplication tables, but they are also making themselves capable to use these skills to solve bunch of numbers. Kids tend to polish their multiplication skills with regular and repetitive practice of multiplication tables. Try to mix these steps or perform each, on a daily basis. The more you practice, the quicker you will polish your multiplication skills.
Math is always unproblematic, if is taught and learned in a correct manner.
HUMAN BRAIN
THE FUNCTIONS OF THE BRAIN
The human brain is a complex organ that allows us to think, move, feel, see, hear, taste, and smell. It controls our body, receives information, analyzes information, and stores information (our memories).
The brain produces electrical signals, which, together with chemical reactions, let the parts of the body communicate. Nerves send these signals throughout the body.
SIZE OF THE HUMAN BRAIN
The average human brain weighs about 3 pounds (1300-1400 g).
At birth, the human brain weighs less than a pound (0.78-0.88 pounds or 350-400 g). As a child grows, the number of cell remains relatively stable, but the cells grow in size and the number of connections increases. The human brain reaches its full size at about 6 years of age.
COMPOSITION OF THE BRAIN
The brain consists of gray matter (40%) and white matter (60%) contained within the skull. Brain cells include neurons and glial cells.
The brain has three main parts: the cerebrum, the cerebellum, and the brain stem (medulla).
NOURISHMENT OF THE BRAIN
Although the brain is only 2% of the body's weight, it uses 20% of the oxygen supply and gets 20% of the blood flow. Blood vessels (arteries, capillaries, and veins) supply the brain with oxygen and nourishment, and take away wastes. If brain cells do not get oxygen for 3 to 5 minutes, they begin to die.
Cerebrospinal fluid (CSF) surrounds the brain.
THE NERVOUS SYSTEM
The brain and spinal cord make up the central nervous system (CNS). The brain is connected to the spinal cord, which runs from the neck to the hip area. The spinal cord carries nerve messages between the brain and the body.
The nerves that connect the CNS to the rest of the body are called the peripheral nervous system.
The autonomic nervous system controls our life support systems that we don't consciously control, like breathing, digesting food, blood circulation, etc.
PROTECTION
The cells of the nervous system are quite fragile and need extensive protection from being crushed, being infected by disease organisms, and other harm. The brain and spinal cord are covered by a tough, translucent membrane, called the dura matter. Cerebrospinal fluid (CSF) is a clear, watery liquid that surrounds the brain and spinal cord, and is also found throughout the ventricle (brain cavities and tunnels). CSF cushions the brain and spinal cord from jolts.
The cranium (the top of the skull) surrounds and protects the brain. The spinal cord is surrounded by vertebrae (hollow spinal bones). Also, some muscles serve to pad and support the spine.
More subtly, the blood-brain barrier protects the brain from chemical intrusion from the rest of the body. Blood flowing into the brain is filtered so that many harmful chemicals cannot enter the brain.
INVENTION OF BULB
The Invention of the Light Bulb: Davy, Swan and Edison
The first electric light was made in 1800 by Humphry Davy, an English scientist. He experimented with electricity and invented an electric battery. When he connected wires to his battery and a piece of carbon, the carbon glowed, producing light. This is called an electric arc.
Much later, in 1860, the English physicist Sir Joseph Wilson Swan (1828-1914) was determined to devise a practical, long-lasting electric light. He found that a carbon paper filament worked well, but burned up quickly. In 1878, he demonstrated his new electric lamps in Newcastle, England.
In 1877, the American Charles Francis Brush manufactured some carbon arcs to light a public square in Cleveland, Ohio, USA. These arcs were used on a few streets, in a few large office buildings, and even some stores. Electric lights were only used by a few people.
The inventor Thomas Alva Edison (in the USA) experimented with thousands of different filaments to find just the right materials to glow well and be long-lasting. In 1879, Edison discovered that a carbon filament in an oxygen-free bulb glowed but did not burn up for 40 hours. Edison eventually produced a bulb that could glow for over 1500 hours.
Lewis Howard Latimer (1848-1928) improved the bulb by inventing a carbon filament (patented in 1881); Latimer was a member of Edison's research team, which was called "Edison's Pioneers." In 1882, Latimer developed and patented a method of manufacturing his carbon filaments.
In 1903, Willis R. Whitney invented a treatment for the filament so that it wouldn't darken the inside of the bulb as it glowed. In 1910, William David Coolidge (1873-1975) invented a tungsten filament which lasted even longer than the older filaments. The incandescent bulb revolutionized the world.
MOLECULAR STRUCTURE




The three dimensional shape or configuration of a molecule is an important characteristic. This shape is dependent on the preferred spatial orientation of covalent bonds to atoms having two or more bonding partners. Three dimensional configurations are best viewed with the aid of models. In order to represent such configurations on a two-dimensional surface (paper, blackboard or screen), we often use perspective drawings in which the direction of a bond is specified by the line connecting the bonded atoms. In most cases the focus of configuration is a carbon atom so the lines specifying bond directions will originate there. As defined in the diagram on the right, a simple straight line represents a bond lying approximately in the surface plane. The two bonds to substituents A in the structure on the left are of this kind. A wedge shaped bond is directed in front of this plane (thick end toward the viewer), as shown by the bond to substituent B; and a hatched bond is directed in back of the plane (away from the viewer), as shown by the bond to substituent D. Some texts and other sources may use a dashed bond in the same manner as we have defined the hatched bond, but this can be confusing because the dashed bond is often used to represent a partial bond (i.e. a covalent bond that is partially formed or partially broken). The following examples make use of this notation, and also illustrate the importance of including non-bonding valence shell electron pairs (colored blue) when viewing such configurations .
Methane Ammonia Water
Bonding configurations are readily predicted by valence-shell electron-pair repulsion theory, commonly referred to as VSEPR in most introductory chemistry texts. This simple model is based on the fact that electrons repel each other, and that it is reasonable to expect that the bonds and non-bonding valence electron pairs associated with a given atom will prefer to be as far apart as possible. The bonding configurations of carbon are easy to remember, since there are only three categories.
Configuration Bonding Partners Bond Angles Example
Tetrahedral 4 109.5º
Trigonal 3 120º
Linear 2 180º
In the three examples shown above, the central atom (carbon) does not have any non-bonding valence electrons; consequently the configuration may be estimated from the number of bonding partners alone. For molecules of water and ammonia, however, the non-bonding electrons must be included in the calculation. In each case there are four regions of electron density associated with the valence shell so that a tetrahedral bond angle is expected. The measured bond angles of these compounds (H2O 104.5º & NH3 107.3º) show that they are closer to being tetrahedral than trigonal or linear. Of course, it is the configuration of atoms (not electrons) that defines the the shape of a molecule, and in this sense ammonia is said to be pyramidal (not tetrahedral). The compound boron trifluoride, BF3, does not have non-bonding valence electrons and the configuration of its atoms is trigonal. Nice treatments of VSEPR theory have been provided by Oxford and Purdue . Click on the university name to visit their site.
The best way to study the three-dimensional shapes of molecules is by using molecular models. Many kinds of model kits are available to students and professional chemists. Some of the useful features of physical models can be approximated by the model viewing plugin Chime.
If this plugin has been installed you may examine several models of compounds discussed above by .
One way in which the shapes of molecules manifest themselves experimentally is through molecular dipole moments. A molecule which has one or more polar covalent bonds may have a dipole moment as a result of the accumulated bond dipoles. In the case of water, we know that the O-H covalent bond is polar, due to the different electronegativities of hydrogen and oxygen. Since there are two O-H bonds in water, their bond dipoles will interact and may result in a molecular dipole which can be measured. The following diagram shows four possible orientations of the O-H bonds.
The bond dipoles are colored magenta and the resulting molecular dipole is colored blue. In the linear configuration (bond angle 180º) the bond dipoles cancel, and the molecular dipole is zero. For other bond angles (120 to 90º) the molecular dipole would vary in size, being largest for the 90º configuration. In a similar manner the configurations of methane (CH4) and carbon dioxide (CO2) may be deduced from their zero molecular dipole moments. Since the bond dipoles have canceled, the configurations of these molecules must be tetrahedral (or square-planar) and linear respectively.
The case of methane provides insight to other arguments that have been used to confirm its tetrahedral configuration. For purposes of discussion we shall consider three other configurations for CH4, square-planar, square-pyramidal and triangular-pyramidal.
Configuration Bonding Partners Bond Angles
Tetrahedral 4 109.5º
Trigonal 3 120º
Linear 2 180º
Substitution of one hydrogen by a chlorine atom gives a CH3Cl compound. Since the tetrahedral, square-planar and square-pyramidal configurations have structurally equivalent hydrogen atoms, they would each give a single substitution product. However, in the trigonal-pyramidal configuration one hydrogen (the apex) is structurally different from the other three (the pyramid base). Substitution in this case should give two different CH3Cl compounds if all the hydrogens react. In the case of disubstitution, the tetrahedral configuration of methane would lead to a single CH2Cl2 product, but the other configurations would give two different CH2Cl2 compounds. These substitution possibilities are shown in the above Chime insert.
VISUAL MERCHANDISE TIPS
Display sale products in a creative way. When building a product display look for products that are natural add-ons to the main product featured. A great visual merchandising tip for a grocery store: Build an end cap special on tomato soup, but don’t just stop with the soup, get creative. Merchandise the entire end cap with tomato soup then place a shelf at eye level displaying brightly colored soup bowls as an add-on sale item. Visually the display would have a repeating label accented by one ribbon shelf of bowls. This is good visual merchandising practice and great way to increase margin.
Use accent lighting to feature products. Customers are drawn to light. Lighting can make products shine and bring colors to life. Using this visual merchandising tip to wash a display wall with light will enhance any product. Accent lighting creates visual interest for shoppers, and magically puts products in their sight. Once a product has captured a customer’s attention the odds are increased that a purchase is at hand. This visual merchandising tip will impact your customers’ impression of their shopping experience and your profits.
Change displays weekly. This visual merchandising tip reminds us that customers want to see new and different products. There is an aspect of entertainment and education that customers appreciate when stores change their displays. Stores that don’t change their displays weekly will have customers simply walking past displays that are no longer fresh. If the customers have seen the same end cap for the last six weeks, the displays are no longer new or relevant to them. These stores will not reap the potential rewards of incremental or add-on sales and can lose out to their competition. If the competitor down the street is constantly and creatively changing their visuals, there is a good chance shoppers will gravitate to that store.
Color matters. Visual merchandising tips like this one are at the core of any solid merchandising plan. Color can demand a shopper’s attention, evoke emotion and influence decisions. Your merchandising plan should include bright colors not only for displays or end caps, but also for the middle of aisle runs. Visually painting your store with colorful focal points will help draw shoppers to those key areas.
Merchandising themes. Consider grouping themed products together for powerful visual messages. Theme merchandising is a fun way to communicate seasonal activities or other information. If your store is a hardware store, use garden gloves, flower bulbs, decorative pots, hand gardening tools, and potting soil to get your sales started for spring. Outdoor grilling displays are great themes for grocery stores and home centers. Backyard chefs will be attracted to every item in your display. Themes connect customers to projects or activities, and as a result, connect their spending to your profits.
SIMPLE BUSINESS PLAN
quantity total sales value average value % gross margin total sales or gross margin
product 1
product 2
product 3
product 4
totals
Do the same for each important aspect of your business, for example, split by market sector (or segment):
quantity total sales value average value % gross margin total sales or gross margin
sector 1
sector 2
sector 3
sector 4
totals
And, for example, split by distributor (or route to market):
quantity total sales value average value % gross margin total sales or gross margin
distributor 1
distributor 2
distributor 3
distributor 4
totals
These simple split analysis tools are an extremely effective way to plan your sales and business. Construct a working spreadsheet so that the bottom-right cell shows the total sales or gross margin, or profit, whatever you need to measure, and by changing the figures within the split (altering the mix, average prices, quantities, etc) you can carry out 'what if?' analysis to develop the best plans.
If you are a competent working with spreadsheets it is normally possible to assemble all of this data onto a single spreadsheet and then show different analyses by sorting and graphing according to different fields.
When you are happy with the overall totals for the year, convert this into a phased monthly plan, with as many lines and columns as you need and are appropriate for the business. Develop this spreadsheet by showing inputs as well as sales outputs - the quantifiable activity (for example, the numbers of enquiries necessary to produce the planned sales levels) required to produce the planned performance. Large businesses need extensive and multiple page spreadsheets. A business plan needs costs as well as sales, and will show profit as well as revenue and gross margin, but the principle is the same: plan the detailed numbers and values of what the business performance will be, and what inputs are required to achieve it.
Here's a free MSExcel profit and loss account template tool for incorporating these factors and financials into a more formal phased business trading plan, which also serves as a business forecasting and reporting tool too. Adapt it to suit your purposes. This plan example is also available as a PDF, see the Profit and Loss Account (P&L) Small Enterprise Business Plan Example (PDF). The numbers could be anything: ten times less, ten times more, a hundred times more - the principle is the same.
Consider also indirect activities that affect sales and business levels, such as customer service. Identify key performance indicators here too, such as customer complaints response and resolution levels and timescales. Internal lead referral schemes, strategic partnership activity; the performance of other direct sales activities such as sales agencies, distributorships, export activities, licensing, etc. These performance factors won't normally appear on a business plan spreadsheet, but a separate plan should be made for them, otherwise they won't happen.
BUSINESS PLAN STRUCTURE
Contents page: A list of contents (basically the sections listed here, starting with the Introduction page) showing page numbers, plus a list of appendices or addendums (added reference material at the back of the document) allowing the reader to find what they need and navigate the document easily, and to refer others to particular items and page numbers when reviewing or querying.
Introduction page: Introduction and purpose of the plan, terms of reference if applicable (usually for formal and large plans or projects).
Executive summary page: Optional and usually beneficial, this should normally be no more than a page long (or it's not an executive summary) - the key points of the whole plan including conclusions, recommendations, actions, financial returns on investment, etc., clearly readable in a few minutes.
Main body of plan: sections and headings as required, see template below.
Acknowledgments and bibliography/reference sources: if relevant (only required normally for very large formal plans)
Appendices: appendices or addendums - additional detailed reference material, examples, statistics, spreadsheets, etc., for reference and not central to the main presentation of your plan.
TYPES OF SNAKES




Snake Pictures Types of Snakes (Non-Venomous) Scientific Name
Brown Water Snake Nerodia taxispilota
Banded Water Snake Nerodia fasciata
Scarlet Snake Cemophora coccinea
Corn Snake Elaphe guttata
Desert Kingsnake Lampropeltis getula splendida
California Kingsnake Lampropeltis getulus californiae
Western Coachwhip Masticophis flagellum flagellum
Rough Green Snake Opheodrys aestivus
Hognose Snake Heterodon platirhinos
Ringneck Snake Diadophis punctatus
Red Sided Garter Snake Thamnophis sirtalis infernalis
Common Garter Snake Thamnophis sirtalis
Ribbon Snake Thamnophis sauritus
Red Milk Snake Lampropeltis triangulum syspila
Gray Banded Kingsnake Lampropeltis alterna
Glossy Snake Arizona elegans occidentalis
Gopher Snake Pituophis melanoleucus catenifer
Anaconda Eunectes murinus
Reticulated Python Python reticulatus
Burmese Python Python molarus bivittatus
The African Rock Python Python sebae sebae
Green Tree Python Morelia viridis
White Lipped Python Leiopython albertisii
Emerald Tree Boa Corallus caninus
Rosy Boa Trivirgata
Yellow Snake (Jamaican boa) Epicrates subflavus
Ball Python Python Regius
King Rat Snake Elaphe carinata
Baird's Rat Snake Elaphe bairdi
Black Rat Snake Elaphe obsoleta
Everglades Rat Snake Elaphe obsoleta rossalleni
Red Tailed Green Rat Snake Elaphe oxycephala
Brown House Snake Lamprophis fuliginosus
Bull snake Pituophis catenifer sayi
Yellow Rat Snake Elaphe Obsoleta Quadrivittata
Mud Snake Farancia abacura
Eastern Indigo Snake Drymarchon couperi
Scarlet Kingsnake Lampropeltis triangulum elapsoides
Black Racer Coluber constrictor
Redbelly Snake Storeria occipitomaculata
Big Bend or New Mexico Milk Snake Lampropeltis triangulum celaenops
Ground Snake Sonora semiannulata
Southwestern Blackhead Snake Southwestern Blackhead Snake
Texas Long-Nosed Snake Rhinocheilus lecontei tessellatus
Trans-Pecos Blind Snake Leptotyphlops humilis segregus
Trans Pecos Rat Snake Bogertophis subocularis
Western Hook-Nosed Snake Gyalopion canum
Gray Rat Snake Elaphe obsoleta spiloides
Mexican Night Snake Pseudelaphe flavirufus
Taiwanese Beauty Rat Snakes Elaphe taeniura
Snake Pictures Types of Snakes (Venomous) Scientific Name
Black Mamba Dendroaspis polylepis
Cobra Dendroaspis angusticeps
King Cobra Snake Ophiophagus Hannah
Cape Cobra Naja nivea
Coral Snake Micrurus fulvius tenere
Copperhead Snake Agkistron contortrix
Mozambique Spitting Cobra Naja mossambica
Egyptian Cobra Naja haje haje
Red Tailed Racer Gonyosoma oxycephala
Eastern Tiger Snake Telescopus semiannulatus
Australian Common Tiger Snake Notechis scutatus
Common Adder Vipera berus
Puff Adder Bitis arietans
Horned Adder Bitis caudalis
Rattlesnake Crotalus lepidus
Diamondback Rattlesnake Crotalus atrox
Timber Rattlesnake Crotalus horridus
Eastern Diamondback Rattlesnake Crotalus adamantus
Black Tailed Rattlesnake Crotalus molossus
Pacific Rattlesnake Crotalus atrox
Mohave Rattlesnake Crotalus scutulatus
Water Moccasin Agkistrodon piscivorus conanti
Fer-de-lance Bothrops asper
Persian Horned Viper Pseudocerastes persicus
Eyelash Viper Bothriechis schlegelii
Temple Pit Viper Tropidolaemus subannulatus
Gaboon Viper Bitis gabonica
Rhinoceros Viper Bitis nasicornis
Godman's Pitviper Porthidium godmani
Philippine Cobra Philippine Cobra
Kraits Krait
Belcher's Sea Snake Hydrophis belch
Australian Copperheads Austrelaps superbus
Red Spitting Cobra Naja pallida
Inland Taipan Oxyuranus microlepidotus
Papuan Taipan Oxyuranus scutellatus canni
Coastal Taipan Oxyuranus scutellatus scutellatus
Eastern Brown Snake Pseudonaja textilis
Mainland Tiger Snake Notechis scutatus scutatus
Peninsula Tiger Snake Notechis ater occidentalis
Chappell Island tiger snake Notechis ater serventyi
King Island/Tasmania Tiger snake Notechis ater humphreysi
Black Headed Death Adder Acanthophis amstrongi
Barkly Tableland Death Adder Acanthophis hawkei
Desert Death Adder Acanthophis pyrrhus
Northern Death Adder Acanthophis praelongus
Gwardar Pseudonaja nuchalis
Bushmaster Lachesis muta muta
Russell’s Viper Vipera russelli
Saw-Scaled Viper Echis carinatus
Rough-Scaled Snake Tropidechis carinatus
Papuan Black Snake Pseudechis papuanus
Multibanded Krait Bungarus Multicinctus
Stephen's Banded Snake Hoplocephalus stephensi
Spotted Black Snake Pseudechis guttatus
Collett's Snake Pseudechis colletti
Mulga Snake Pseudechis australis
Red-bellied Black Snake Pseudechis porphyriacus
Small-Eyed Snake Cryptophis nigrescens
Marbled Headed Whip Snake Demansi olivacea
Barba Amarilla Bothrops atrox
Horned Sea Snake Acalyptophis peronii
Highland Copperhead Austrelaps labialis
Pygmy Copperhead Austrelaps ramsayi
Lake Cronin Snake Echiopsis atriceps
Bardick Snake Echiopsis curta
Pale-Headed Snake Hoplocephalus bitorquatus
Broad-Headed Snake Hoplocephalus bungaroides
Jararacussu Bothrops jararacussu
Butler's Snake/Spotted Mulga Pseudechis butleri
Speckled Brown Snake Pseudonaja guttata
Peninsula Brown Snake Pseudonaja inframacula
Ringed Brown Snake Pseudonaja modesta
TOTAL SPECIES IN WORLD
The greatest diversity exists among insects, which account for nearly a million of the planet's species. Mammals make up one of the smallest groups, with just 5,416 members. Altogether the earth's oceans and continents support close to 60,000 species of vertebrate animals and 300,000 species of plants.
Total number of species known to exist now in the world.
Category Species Totals
Vertebrate Animals
Mammals 5,416
Birds 9,956
Reptiles 8,240
Amphibians 6,199
Fishes 30,000
Total Vertebrates 59,811
Invertebrate Animals
Insects 950,000
Molluscs 81,000
Crustaceans 40,000
Corals 2,175
Others 130,200
Total Invertebrates 1,203,375
Plants
Flowering plants (angiosperms) 258,650
Conifers (gymnosperms) 980
Ferns and horsetails 13,025
Mosses 15,000
Red and green algae 9,671
Total Plants 297,326
Others
Lichens 10,000
Mushrooms 16,000
Brown algae 2,849
Total Others 28,849
TOTAL SPECIES 1,589,361
FACT OF HUMAB BODY
Nerve impulses to and from the brain travel as fast as 170 miles (274 km) per hour.
The thyroid cartilage is more commonly known as the adams apple.
The only jointless bone in your body is the hyoid bone in your throat
It's impossible to sneeze with your eyes open.
Your stomach needs to produce a new layer of mucus every two weeks or it would digest itself.
It takes the interaction of 72 different muscles to produce human speech.
The average life of a taste bud is 10 days.
The average cough comes out of your mouth at 60 miles (96.5 km) per hour.
Relative to size, the strongest muscle in the body is the tongue.
Human thigh bones are stronger than concrete.
When you sneeze, all your bodily functions stop even your heart.
Babies are born without knee caps. They don't appear until the child reaches 2-6 years of age.
Children grow faster in the springtime.
It takes the stomach an hour to break down cow milk.
Women blink nearly twice as much as men.
Blondes have more hair than dark-haired people do.
There are 10 human body parts that are only 3 letters long (eye hip arm leg ear toe jaw rib lip gum).
If you go blind in one eye you only lose about one fifth of your vision but all your sense of depth.
The average human head weighs about 8 pounds.
Our eyes are always the same size from birth, but our nose and ears never stop growing.
In the average lifetime, a person will walk the equivalent of 5 times around the equator.
An average human scalp has 100,000 hairs.
The length of the finger dictates how fast the fingernail grows. Therefore, the nail on your middle finger grows the fastest, and on average, your toenails grow twice as slow as your fingernails.
The average human blinks their eyes 6,205,000 times each year.
The entire length of all the eyelashes shed by a human in their life is over 98 feet (30 m).
Your skull is made up of 29 different bones.
Your ears and nose continue to grow throughout your entire life.
After you die, your body starts to dry out creating the illusion that your hair and nails are still growing after death.
Hair is made from the same substance as fingernails.
The average surface of the human intestine is 656 square feet (200 m).
A healthy adult can draw in about 200 to 300 cubic inches (3.3 to 4.9 liters) of air at a single breath, but at rest only about 5% of this volume is used.
The surface of the human skin is 6.5 square feet (2m).
15 million blood cells are destroyed in the human body every second.
The pancreas produces Insulin.
The most sensitive cluster of nerves is at the base of the spine.
The human body is comprised of 80% water.
The average human will shed 40 pounds of skin in a lifetime.
Every year about 98% of the atoms in your body are replaced.
The human heart creates enough pressure to squirt blood 30 feet (9 m).
You were born with 300 bones. When you get to be an adult, you have 206.
Human thighbones are stronger than concrete.
Every human spent about half an hour as a single cell.
There are 45 miles (72 km) of nerves in the skin of a human being.
The average human heart will beat 3,000 million times in its lifetime and pump 48 million gallons of blood.
Each square inch (2.5 cm) of human skin consists of 20 feet (6 m) of blood vessels.
During a 24-hour period, the average human will breathe 23,040 times.
Human blood travels 60,000 miles (96,540 km) per day on its journey through the body.
EARTH SEVEN LAYERS
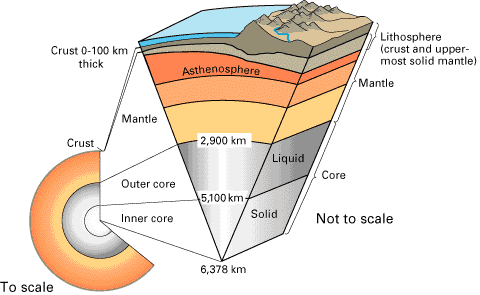
When scientists started to probe the valleys of Earth and in order to know its structure and constituents, they found that tales and myths that dominated in the earlier centuries have no scientific basis. After scientists discovered that the earth is egg-shaped , they suggested that the core of this ball has a nucleus, and its shell is a very thin crust when compared to the Earth’s size, and between these two layers, there is a third, known as the mantle . this was the knowledge of the early scientists.
Development of Scientific Facts
The Three Layer -Theory did not last for so long due to recent discoveries in Geology. Recent measurements and experiments showed that the material comprising the nucleus of the Earth is under very high pressure, three million times more than that on the surface of the Earth.
Under such pressure, matter transforms into solid state, this in turn makes Earth’s core very solid,This core is surrounded by a liquid layer of very high temperature. This means that there are two layers in Earth’s core and not one.Two layers; one solid in the center surrounded by another liquid layer.
Thereafter measuring devices advanced and presented to scientists a clear distinction among Earth’s inner layers. If we were to descend under the earth’s crust we would find another layer of very hot stones , which is the stone cover or wrap. After that come three other distinct layers of varying density, pressure and temperature.
Therefore scientists found themselves classifying the layers of Earth into seven layers, not more. The figure shows these layers with their dimensions (some are out of scale) according to what scientists have recently found using methods like earthquake measuring devices and the study of Earth’s magnetic field, among other techniques. These studies and discoveries are being taught to these scientists’ students in universities.
Figure showing Earth’s seven layers, notice that the crust is very thin followed by mantle of varying thickness, then came the core comprised of a liquid and finally a seventh solid nucleus.
Scientists have found that the atom is also comprised of seven layers or levels, and this proves the uniformity of creation, where Earth has seven layers and atoms have seven layers as well. This creation therefore is ruled by only one creator.
Earth’s seven layers vary greatly in structure, density, temperature and material. Therefore one cannot consider that Earth has only one layer as people anciently thought. Here we find that the idea of the Earth having layers is fairly recent and wasn’t presented or brought forward at the time when the Quran was being revealed. Those discoveries are what the Twenty First century -scientists tell us, What then does the book of Allah tell us?
In the Company of the Noble Quran
The Holy Quran, God's word , talks to us about the seven Heavens and also seven layers of Earth in the Quranic verses:
God says in the Holy Quran in this regard what means “Who has created the seven heavens one above another…” [Al-Mulk 3]
God says in the Holy Quran in this regard what means “It is Allah Who has created seven heavens and of the earth the like thereof (i.e. seven).” [Attalaq 12
The first verse has specified two traits for the heavens which are: their number which is seven, their organization, which is into layers , one on the of top of the other and this is according to the Quranic interpretation books and Arabic language dictionaries. As for the second verse, it confirms that Earth is similar to the Heavens from the part of the verse that says “…and of the earth the like thereof”, where since the Heavens are layers, then Earth is comprised of layers as well, and since the Heavens are seven, therefore Earth’s layers are seven as well.
Here we need to pause and elaborate on Allah’s choice of words in the word: “Tibaqa” – which means layers one on top of another, and this is what scientists have recently discovered that Earth is comprised of layers, this in turn leads us to conclude that Al-Qur`an defined Earth’s shape, of layers, and it (Al-Quran) has also specified the number of these layers, seven. Henceforth Al-Qur`an chose a very specific word to describe Earth’s composition, so Al-Qur`an preceded the Twenty First Century scientists in mentioning Earths facts fourteen centuries ago. Isn’t this an overwhelming Quranic miracle?
If we contemplate 0n the Noble Prophet’s (peace and blessings be upon him) Hadeeth (sayings), we find one Hadeeth that confirms this fact of Earth’s seven overlapping layers. Prophet Mohammad (peace and blessings be upon him) says: “whomever oppressed someone a hand span’s distance from the Earth, gets surrounded by seven overlapping layers”[Bukhari 2321], the Hadeeth is telling us that Allah will punish those who oppress in Earth by being encircled and surrounded by seven Earthly layers. This definition for the word “Tawaqa” that was used in the Hadeeth is as found in Arabic Language Dictionaries.
The question here is: Isn’t this a great prophetic miracle? doesn’t this Hadeeth specify the number of Earth’s layers which are seven, and also mentions their nature of being overlapped or surrounding each other?From the word encircled used in the Hadeeth, we can deduce circular shapes and in three dimensions we can deduce spherical shapes.
Henceforth The Noble Qur’an and Prophetic Sunnah (traditions of the Prophet) preceded modern science to this scientific fact. In addition Al-Qur’an gave us the precise naming for the structure of Earth using the word “Tibaqa” or layers, and it has given us the accurate number for these layers which is seven, while scientists spent long years and changed their theories several times to come up with scientific theories previously mentioned in The Quran and Sunnah. Glory be to Allah the Greatest who said in his exalted book: “And on the earth are signs for those who have Faith with certainty, And also in your ownselves. Will you not then see?” [Athariyat, 20,21]
AREA OF A SECTOR
On this page we'll show you how to find the arc length a and the area A of the sector.
In both calculations we'll need to know the radius r of the circle, and the central angle of the sector.
Finding Arc Length
We'll use a circle with radius 15 cm and central angle 60°.
The first step is to find out what fraction of the circle
is represented by the central angle 60°:
The sector represents 1/6 of the circle,
or 0.1666... of the circle.
To find the arc length a, we now need to find the circumference (the distance around the full circle), and then find 1/6 of it (multiply it by 0.1666...)
This is the length of the arc a.
This method will work for any sector in any circle. You just need to find the fraction of the circle that the sector angle represents, and then find that fraction of the circumference.
Here's a quicker formula to use, that summarizes what we did in one calculation.
Note: Math 30 students will learn a new formula for finding arc length: a = r , where is measured in radians.
Finding the Sector Area
Again we'll use a circle with radius 15 cm and central angle 60°.
The first step once again is to find out what fraction of the circle
is represented by the central angle 60°:
The sector represents 1/6 of the circle,
or 0.1666... of the circle.
To find the sector area A, we now need to find the area of the full circle, and then find 1/6 of it (multiply it by 0.1666...)
This is the area of the sector, A.
This method will work for any sector in any circle. You just need to find the fraction of the circle that the sector angle represents, and then find that fraction of the area.
Here's a quicker formula to use, that summarizes what we did in one calculation.
AREA OF CIRCLE
Area of the circle
Figure out the length of the radius. This may be as simple as dividing the diameter by 2.
Note the formula. The formula for finding the area of a circle is:
Multiply the radius by itself to square it.
Multiply by pi.
If the instructions say "leave in terms of pi", then just stick the pi onto your number.
If the instructions say anything about rounding, replace pi with 3.14 or use your calculator's pi button.
Here is an example:
Occasionally you will see a circle inside of a square. The side length of the square matches the diameter of the circle.
Occasionally you may see a square inside of a circle. The diagonal of the square is also the diameter of the circle.
ORACLE
New Features and Java Server Faces Components Boost Developer Productivity
ORACLE OPENWORLD , SAN FRANCISCO Designed to meet a broad range of developer requirements and skill sets, Oracle today announced, Oracle JDeveloper 10g Release 2. The new release features a brand new look, smaller footprint and scores of developer productivity enhancements. In addition, Oracle is announcing early availability of Oracle Application Development Framework (ADF) Faces, a rich set of graphical user interface components that implement the JavaServer Faces standard and improve productivity for Java developers building Web interfaces.
For Java developers who are primarily focused on coding Java applications, Oracle JDeveloper 10g Release 2 features enhanced Java editing, compiling, and deployment, as well as XML coding features like an XML Editor, XML Schema Editor and XSLT Mapping Tools. Advanced code navigation, hierarchy browsing, code refactoring and code templating features all help streamline the development process. Oracle JDeveloper 10g Release 2 also includes major enhancements to support team development including more seamless integration with Concurrent Versions System (CVS) and Clearcase for source code management, shareable project files and collaborative development facilities.
For the J2EE developer, Oracle JDeveloper 10g Release 2 also provides enterprise-class support for Web services, relational database access and object persistence, the Struts framework, and Web application development. Integration with the award-winning Oracle TopLink Java Persistence Architecture is a key component, enabling enterprise developers to simplify data access and integration of Java objects with any JDBC compliant database.
Professional developers of all skill levels can take advantage of Oracle JDeveloper's enhanced Application Development Framework (ADF), a J2EE productivity layer that simplifies application development by implementing J2EE and Service Oriented Architecture best practices as a set of pre-built runtime components. Architected with 100 percent open Java and Web services interfaces, Oracle ADF speeds development, improves code reuse, and deploys to any J2EE server. "Application development is not a black and white process as the needs and requirements of developers vary greatly," said Ted Farrell, chief architect, Oracle Application Development Tools, Oracle Corp.
"Oracle JDeveloper 10g provides developers with a comprehensive offering for all skill sets, with a J2EE development framework that allows newer Java developers to quickly become productive while minimizing tedious low-level coding for more experienced developers."
Rich Support for Developing Standards-based User Interfaces
Illustrating its continuing support of open standards-based interoperability, Oracle has developed ADF Faces, based on the JavaServer Faces (JSR-127) specification. ADF Faces speeds application development by providing a set of user-interface components that can be easily customized and re-used, ensuring a consistent look and feel while allowing developers to focus on simply designing the user interaction and application flow.
Oracle ADF Faces can be used in any Integrated Development Environment that supports JavaServer Faces. Many of the framework features most needed by JavaServer Faces developers are available now and include, integrated file upload support, automatic client-side validation, a page flow scope that makes it easier to pass values from one page to another, and a hybrid state saving strategy that gives developers the best of both client and server-side state saving.
Additional information on Oracle JDeveloper 10g Release 2 can be found at: http://www.oracle.com/technology/products/jdev/index.html.
About Oracle JDeveloper 10g
With full support for open-standards development on numerous operating systems, Oracle JDeveloper 10g provides complete development life-cycle support for Java developers creating J2EE applications and Web services. Oracle JDeveloper 10g offers built-in features for optimizing the performance of Java applications while providing a single Integrated Development Environment for Java, XML and SQL; business intelligence; UML modeling and J2EE Web services.
About Oracle
Oracle (NASDAQ: ORCL) is the world's largest enterprise software company. For more information about Oracle, visit our Web site at http://www.oracle.com.
Laptop is an essential gadget for every group of people like the businessmen, students, bloggers, BPO workers and there are different types of laptops like notebooks, tablet PCs, netbooks to suit their needs. Getting a good performing laptop involves investing a handsome amount of money and cheap laptops sometimes does not serve the purpose. Here we have made a list of 5 laptops in Indian market that will give you the best bang for your buck. The list includes :
1. Dell Inspiron 1525
2. Acer Aspire 4930
3. Lenovo IdeaPad Y330
4. LG R510
5. Sony Vaio CS
Read our article on top 5 things to consider before buying a laptop if you can’t decide which one to buy.
1. Dell Inspiron 1525
It has the best price to performance ratio with all the features and also an HDMI port.
Display : 15.4 inch WXGA + (1440×900 pixels) resolution
RAM : 2GB DDR2 (1024MB x 2, 667MHz) Dual Channel Support, Up to 4GB support)
Hard Disk : 160 GB SATA, 5400 RPM
Battery : 9- Cell 85WHr Lithium-ion battery
Processor : Intel Core 2 Duo T7250 2.0 GHz 2 MB L2 Cache, 800 MHz FSB
Weight (Kgs) : Approx. 2.70 Kg
Price
Rs. 37900
2. Acer Aspire 4930
This notebook has a good sturdy build and stylish design but battery life is not good.
Screen Size : 14.1 inch WXGA+ Wide TFT display WXGA (1280x 800)
Processor : Intel Core2Duo Processor T5800 2 GHz, 2 MB Cache, 800MHz FSB
Optical Drive : Dual Layer DVDRW
Hard Disk : 160 GB SATA, 5400RPM
Memory : 2GB DDR2 (667 MHz ) Dual channel mode
Weight (Kgs) : 2.4 Kgs
Operating System : Microsoft Windows Vista Home Premium
Battery : 6 cell battery
Price
Rs. 33000
3. Lenovo IdeaPad Y330
The laptop boosts high processing power with ATI graphics and slot loading drive with eject.
Processor : Intel® Pentium® Dual Core T3200 processor ( 2.00GHz 667MHz 1MB )
Operating system : Genuine Windows Vista Home Premium
System graphics : Intel Graphics Media Accelerator 4500MHD
Total memory : 2 GB PC2-5300 DDR2 SDRAM 667MHz
Display type : 13.3 ” WXGA LED Backlight TFT 1280×800
Hard drive device : 250GB 5400
Optical device : DVD Recordable (Dual Layer)
Battery : 6 Cell Lithium-Ion
Price
Rs. 40990
4. LG R510
The notebook has a good sturdy build and design with large 15.4 inch screen but the white keys may not be for everyone.
Display : 15.4″ LED WXGA(1280×800)*Fine Bright Technology
Operating System : Genuine Windows Vista Home Basic
HDD : 250GB(2.5″ 5400rpm SATA)
Processor : Intel Pentium Dual-Core Processor T3200(2.0GHz), 667MHz FSB, 1MB L2 Cache
Memory : 2GB DDR2 (800MHz)
Graphics : Mobile Intel GMA 4500MHD
Weight (Kgs) : 2.70 kg(6cell & ODD) / 2.55 kg (6cell & weight saver)
Price
Rs. 36900
5. Sony VAIO CS - VGN-CS12GH/B
Most of the Vaio Laptops are high priced but this one may fit your budget.
Processor : Intel® Core™2 Duo Processor T5800 (2 GHz)*1*2
Hard Disk Drive : 160 GB (Serial ATA, 5400 rpm)
RAM : 1 GB DDR2 SDRAM
DVD±RW/±R DL/RAM Drive
Display : 14.1 (35.8 cm) wide (WXGA:1280 x 800) TFT Colour display (Clear Bright LCD Lite)
Operating System : Genuine Windows Vista® Home Basic (English Version) with Service Pack 1
Price
Rs. 44990
Apple cites suppliers for violations
In 2009, according to its 2010 Progress Report (PDF), Apple's audits of suppliers turned up 17 core violations, those that it considers to be the most serious practices or situations that run counter to the central principles of the company's Supplier Code of Conduct.
Of those core violations, three involved the hiring of underage workers; three involved the use of noncertified vendors for the disposal of hazardous waste; three involved the presentation of falsified records during the audit; and eight involved excessive recruitment fees.
The supplier code of conduct and the site audits are intended to protect workers' rights and to improve factory conditions.
Apple did not identify any suppliers by name or the countries in which the infractions took place.
In the case of the underage labor, three facilities had hired 15-year-olds in countries where the minimum employment age is 16. In addition, Apple said it found records of 11 workers who had been hired before reaching that minimum age, though those people were no longer underage or were no longer actively employed at the facilities.
Following up its investigation of how those people had come to be hired, Apple said it required the facilities to protect against future such violations through more thorough ID checks and verification procedures.
In the case of the hazardous waste disposal, Apple required the three facilities to stop shipping waste, to hire certified vendors, and to bring on an outside consultant for a thorough review of the systems used in managing hazardous wastes. It also prompted the facilities to immediately perform inspections of their wastewater disposal systems.
The suppliers who provided falsified records included one attempt to conceal evidence of historical instances of underage workers, and two attempts to conceal violations regarding working hours and days of rest.
As for the excessive recruitment fees discovered, Apple required suppliers to reimburse fees exceeding set limits. Foreign workers, Apple said, have been reimbursed $2.2 million in recruitment fee overcharges over the past two years.
In 2009, Apple conducted on-site audits of 102 facilities. That included annual audits of all final assembly manufacturers (which assemble products including Macs and iPhones), first-time audits of component and nonproduction suppliers (which deliver goods including LCDs, hard drives, keyboards, as well as office supplies), and 15 repeat audits at facilities where it had earlier found instances of core violations.
"In general," Apple said in the report, "annual audits of final assembly manufacturers show continued performance improvements and better working conditions."
Apple said that, in total, more than 133,000 workers, supervisors, and managers have been trained on matters of workers' rights and management's responsibilities.
A Multiplication Table
|
|
|
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
Multiplication Table
| x | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| 2 | 0 | 2 | 4 | 6 | 8 | 10 | 12 | 14 | 16 | 18 | 20 | 22 | 24 |
| 3 | 0 | 3 | 6 | 9 | 12 | 15 | 18 | 21 | 24 | 27 | 30 | 33 | 36 |
| 4 | 0 | 4 | 8 | 12 | 16 | 20 | 24 | 28 | 32 | 36 | 40 | 44 | 48 |
| 5 | 0 | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 | 45 | 50 | 55 | 60 |
| 6 | 0 | 6 | 12 | 18 | 24 | 30 | 36 | 42 | 48 | 54 | 60 | 66 | 72 |
| 7 | 0 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | 56 | 63 | 70 | 77 | 84 |
| 8 | 0 | 8 | 16 | 24 | 32 | 40 | 48 | 56 | 64 | 72 | 80 | 88 | 96 |
| 9 | 0 | 9 | 18 | 27 | 36 | 45 | 54 | 63 | 72 | 81 | 90 | 99 | 108 |
| 10 | 0 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | 100 | 110 | 120 |
| 11 | 0 | 11 | 22 | 33 | 44 | 55 | 66 | 77 | 88 | 99 | 110 | 121 | 132 |
| 12 | 0 | 12 | 24 | 36 | 48 | 60 | 72 | 84 | 96 | 108 | 120 | 132 | 144 |
Remembering 9's
What's 9 x 7 ? Use the 9-method! Hold out all 10 fingers, and lower the 7th finger. There are 6 fingers to the left and 3 fingers on the right.
The answer is 63!
Types of Mountains
How are mountains formed? Mountains are formed by slow but gigantic movements of the earth's crust (the outer layer of the Earth). The Earth's crust is made up of 6 huge slabs called plates, which fit together like a jigsaw puzzle. When two slabs of the earth's crust smash into each other the land can be pushed upwards, forming mountains. Many of the greatest mountain ranges of the world have formed because of enormous collisions between continents. Sometimes the crust has folded and buckled, sometimes it breaks into huge blocks. In both cases, great areas of land are lifted upwards to form mountains. Other mountains are formed by the earth's crust rising into a dome, or by volcanic activity when the crust cracks open.Mountains form in different ways What different types of Mountains are there? There are five basic kinds of mountains:
Fold mountains are the most common type of mountain. The world’s largest mountain ranges are fold mountains. These ranges were formed over millions of years. Fold mountains are formed when two plates collide head on, and their edges crumbled, much the same way as a piece of paper folds when pushed together.
The upward folds are known as anticlines, and the downward folds are synclines. Examples of fold mountains include:
The Himalayan Mountains were formed when India crashed into Asia and pushed up the tallest mountain range on the continents. In South America, the Andes Mountains were formed by the collision of the South American continental plate and the oceanic
Instead of the earth folding over, the earth's crust fractures (pulls apart). It breaks up into blocks or chunks. Sometimes these blocks of rock move up and down, as they move apart and blocks of rock end up being stacked on one another.
Often fault-block mountains have a steep front side and a sloping back side. Examples of fault-block mountains include:
As the dome is higher than its surroundings, erosion by wind and rain occurs from the top. This
As the name suggests, volcanic mountains are formed by volcanoes. Volcanic Mountains are formed when molten rock (magma) deep within the earth, erupts, and piles upon the surface. Magna is called lava when it breaks through the earth's crust. When the ash and lava cools, it builds a cone of rock. Rock and lava pile up, layer on top of layer.
Examples of volcanic mountains include:
Plateau mountains are not formed by internal activity. Instead, these mountains are formed by erosion. Plateaus are large flat areas that have been pushed above sea level by forces within the Earth, or have been formed by layers of lava. The dictionary describes these as large areas of ‘high levels’ of flat land, over 600 meters above Plateau mountains are often found near folded mountains. As years pass, streams and rivers erode valleys through the plateau, leaving mountains standing between the valleys. The mountains in New Zealand are examples of plateau mountains |





 Dome mountains are the result of a great amount of melted rock (magna) pushing its way up under the earth crust. Without actually erupting onto the surface, the magma pushes up overlaying rock layers. At some point, the magma cools and forms hardened rock. The uplifted area created by rising magma is called a dome because of looking like the top half of a sphere (ball). The rock layers over the hardened magma are warped upward to form the dome. But the rock layers of the surrounding area remain flat.
Dome mountains are the result of a great amount of melted rock (magna) pushing its way up under the earth crust. Without actually erupting onto the surface, the magma pushes up overlaying rock layers. At some point, the magma cools and forms hardened rock. The uplifted area created by rising magma is called a dome because of looking like the top half of a sphere (ball). The rock layers over the hardened magma are warped upward to form the dome. But the rock layers of the surrounding area remain flat.
 Volcanic Mountains
Volcanic Mountains